Finding Probabilities For Normal Distributions

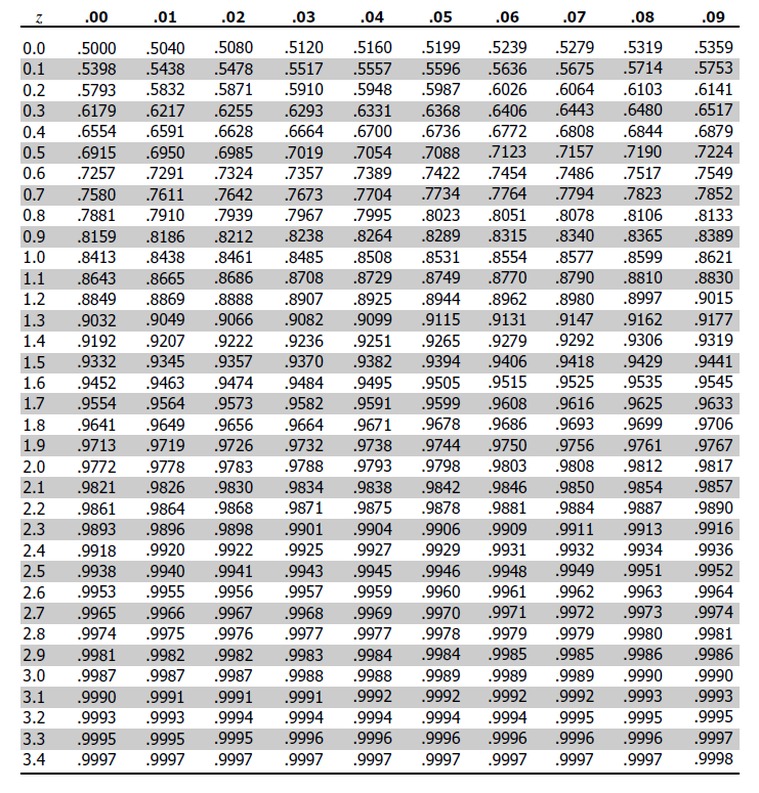
Using the standard normal distribution table, nosotros can confirm that a ordinarily distributed random variable $Z$, with a hateful equal to 0 and variance equal to ane, is less than or equal to $z$, i.e., $P(Z ≤ z)$.

Even so, the table does this only when we have positive values of $z$. Merely put, if an examiner asks you to detect the probability behind a given positive z-value, you lot will have to look it upward directly on the tabular array knowing that $P(Z ≤ z) = θ(z)$ when $z$ is positive.

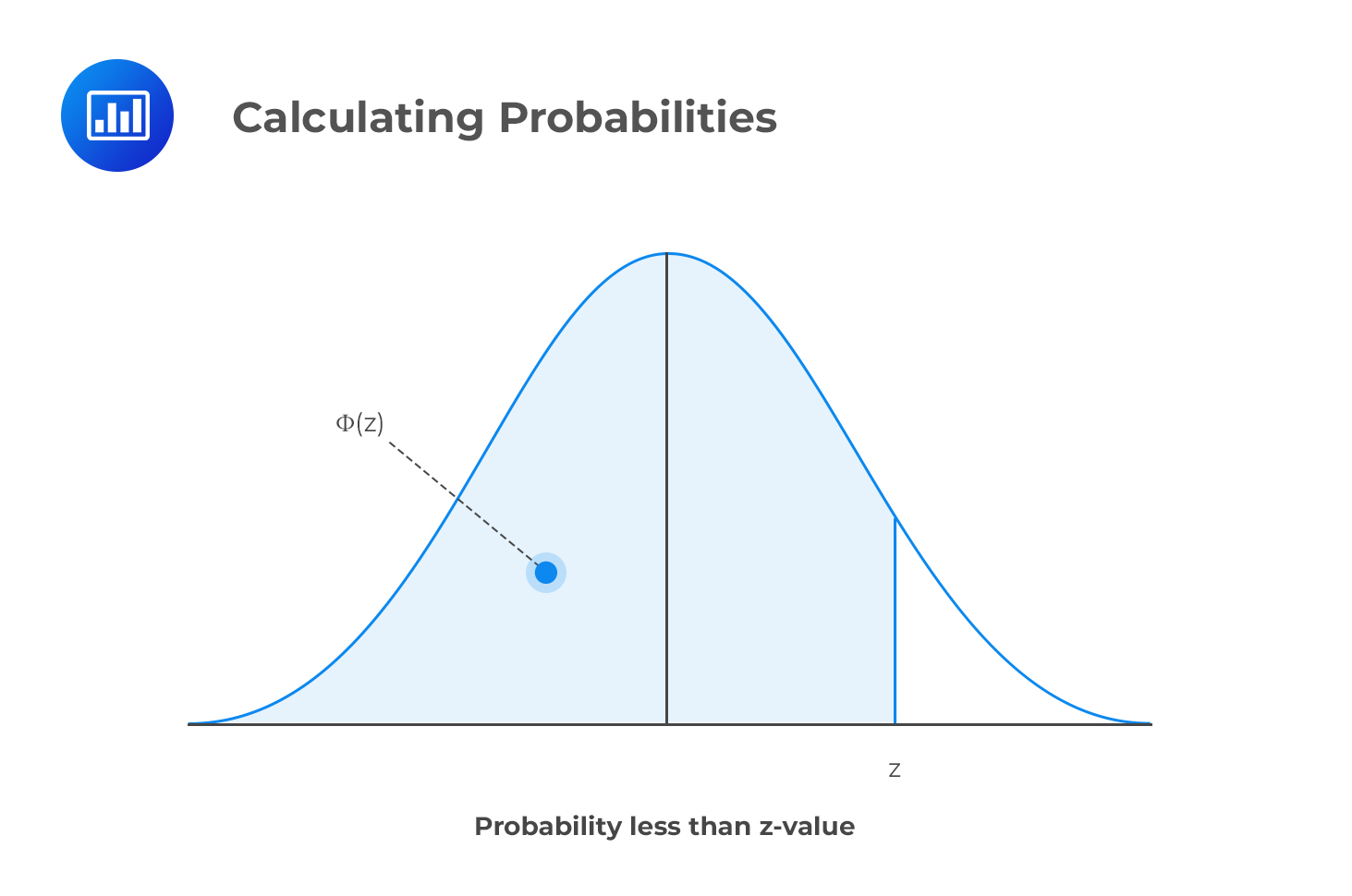
calculating-probabilities

Positive z-values

Example: Using the z-score Tabular array

The returns on ABC stock are normally distributed where the mean is $0.threescore with a standard difference of $0.20. The probability that the return is less than $1 is closest to:

Solution

If the render is $0.x, and then $x = 0.one$ (this is our observed value). Therefore,

$$\begin{align} z &=\frac{10-\mu}{\sigma}\\&=\frac{1-0.6}{0.2}\\ &=2\ \text{(The render of \$1 is two and a half standard deviations below the hateful)}\stop{align}$$

First, you lot'd be required to summate the z-value (2 in this case). $P(Z ≤ 1)$ can be read off directly from the table

You only move down and locate the z-value that lies to the correct of "two," i.e., 0.9772.

z-table-positive

Negative z-values

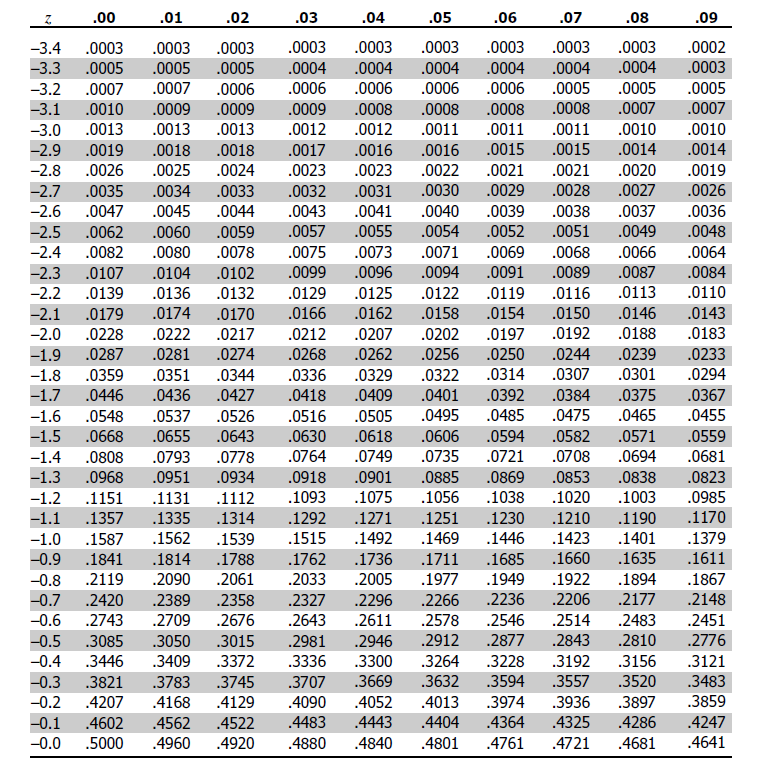
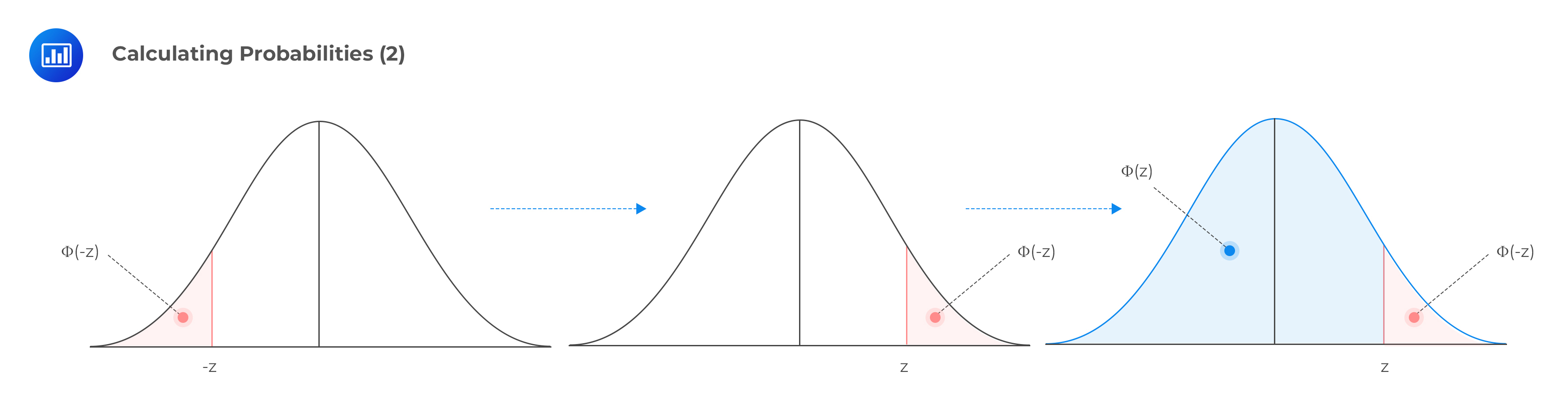
If we have a negative z-value and do not have admission to the negative values from the table (equally shown below), we can nonetheless calculate the corresponding probability by noting that:

$$ \brainstorm{align} P(Z \le -z) & = i – P(Z \le z) \\ \theta(–z) & = 1 – \theta(z) \\ 0.0228 & = 1 – 0.9772 \stop{align}$$

z-table

This relationship is true when we consider the post-obit facts:

The full area (probability) under the standard normal distribution is ane.
The standardized normal distribution is symmetrical well-nigh the mean.

calculating-probabilities-2

Question

Calculate P(Z ≤ -two.v).

A. 0.9938

B. 0.0062

C. 0.06

Solution

The right reply is B.

$$ \begin{marshal*} P(Z \le -two.5) & = 1 – P(Z \le 2.5) \\ & = 1 – 0.9938 \\ & = 0.0062 \\ \terminate{align*} $$